Avatar

Marek Kaluba

Mathematician

Freelance

Biography

Between 2021 and 2024 I was an independent researcher at Karlsruher Institute für Technologie associated to the Topology group led by Roman Sauer.

I was an assistant professor at the Adam Mickiewicz University from 2014 until 2021.

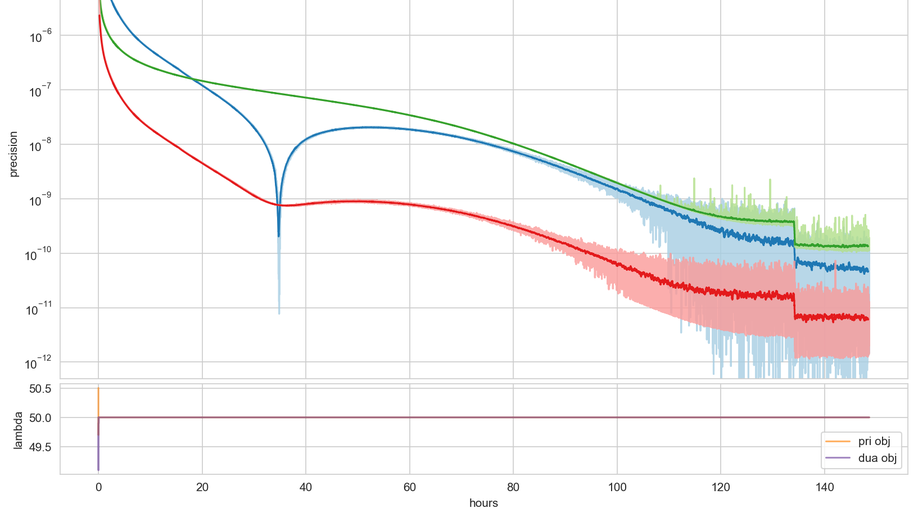
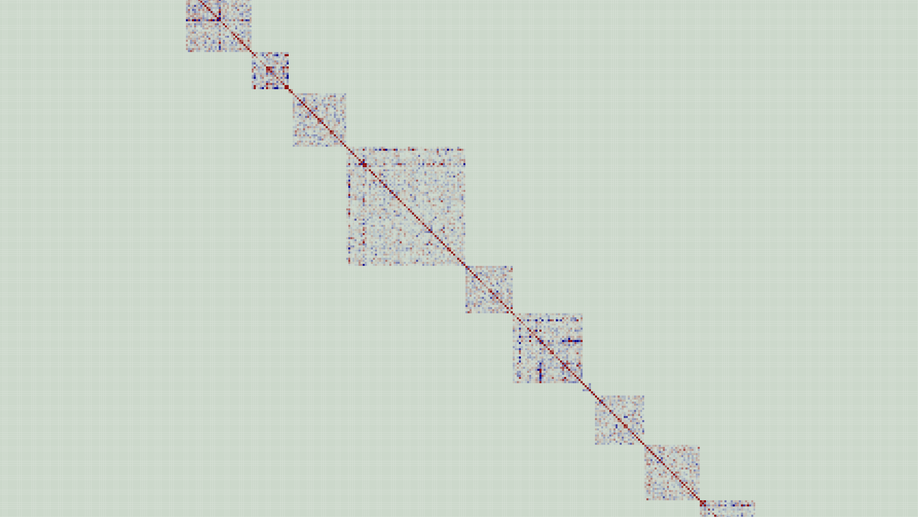
The Mathematical Institute of the Polish Academy of Sciences hosted me as visiting professor between 2015 and 2017, where I worked with Piotr Nowak on Kazhdan’s property (T).

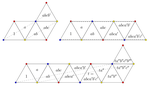
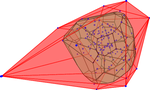
Between 2019 and 2021 I was a postdoc in the MATH+ programm hosted at Technische Universität Berlin in the group of Michael Joswig, where I was working on machine learning aspects of polytope theory.

My research interests also include: geometric group theory (therefore: group actions), optimisation as well as symbolic and certified computation. Before, I used to work on topology of high-dimensional manifolds (surgery and equivariant surgery theory) and applied topology (persistence and others). An important part of my research has become programming – the effects may be found on github.

Programming languages: Julia, Python

Sport: Climbing, Yoga

Interests

  • Computational Algebra
  • Property (T)
  • Geometric Group Theory

Education

  • PhD in Mathematics, 2014

    Adam Mickiewicz University

  • MSc in Mathematics, 2010

    Adam Mickiewicz University

Featured Publications

December 2018 Ann. of Math. (2021) 193: 539-562

On property (T) for $\operatorname{Aut}(F_n)$ and $\operatorname{SL}_n(\mathbb{Z})$

We prove that $\operatorname{Aut}(F_n)$ has Kazhdan’s property (T) for every $n \geqslant 6$. Together with the previous result of Kaluba, Nowak, and Ozawa, this gives the same statement for $n \geqslant 5$. We also provide new, explicit lower bounds for the Kazhdan constants of $\operatorname{SAut}(F_n)$ (with $n \geqslant 6$) and of $\operatorname{SL}_n(\mathbb{Z})$ (with $n \geqslant 3$) with respect to natural generating sets. In the latter case, these bounds improve upon previously known lower bounds whenever $n > 6$.

Code Dataset Slides DOI ArXiv ipynb
December 2017 Math. Ann. (2019) 375: 1169-1191

$\operatorname{Aut}(\mathbb{F}_5)$ has property (T)

We prove that $\operatorname{Aut}(\mathbb{F}_5)$ has Kazhdan’s property (T).

PDF Code Dataset Slides DOI ArXiv Nextjournal

Recent Publications

Quickly discover relevant content by filtering publications.

Hyperbolic generalized triangle groups, property (T) and finite simple quotients

We investigate generalized triangle groups in search for both hyperbolicity and property (T).
Code Slides ArXiv

Geometric Disentanglement by Random Convex Polytopes

We propose a new geometric method for measuring the quality of representations obtained from deep learning. Our approach, called Random …
Project Poster ArXiv

Polymake.jl: A new interface to polymake

A novel julia interface to polymake.
PDF Code Project Slides Video DOI ArXiv

On property (T) for $\operatorname{Aut}(F_n)$ and $\operatorname{SL}_n(\mathbb{Z})$

We prove that $\operatorname{Aut}(F_n)$ has Kazhdan’s property (T) for every $n \geqslant 6$. Together with the previous result of Kaluba, Nowak, and Ozawa, this gives the same statement for $n \geqslant 5$. We also provide new, explicit lower bounds for the Kazhdan constants of $\operatorname{SAut}(F_n)$ (with $n \geqslant 6$) and of $\operatorname{SL}_n(\mathbb{Z})$ (with $n \geqslant 3$) with respect to natural generating sets. In the latter case, these bounds improve upon previously known lower bounds whenever $n > 6$.
Code Dataset Slides DOI ArXiv ipynb

$\operatorname{Aut}(\mathbb{F}_5)$ has property (T)

We prove that $\operatorname{Aut}(\mathbb{F}_5)$ has Kazhdan’s property (T).
PDF Code Dataset Slides DOI ArXiv Nextjournal
See all publications

Experience

 
 
 
 
 

Postdoc

Karlsruher Institute für Technologie

Apr 2021 – Present Karlsruhe, Germany
 
 
 
 
 

MATH+ postdoc

Technische Universität Berlin

Feb 2019 – Mar 2021 Berlin, Germany
Approximate Convex Hulls With Bounded Complexity
 
 
 
 
 

Assistant Professor

Mathematical Institute of Polish Academy of Sciences

Oct 2015 – Nov 2017 Warsaw, Poland
Computational aspects of Kazhdan’s property (T)
 
 
 
 
 

Assistant Professor

Faculty of Mathematics and Computer Science of AMU

Oct 2014 – Feb 2021 Poznań, Poland

Upcoming Talks

Past talks

Recent Posts

SCS on gpu in julia

Splitting Conic Solver or scs is a well established solver for conic optimization problems. It has bindings to julia via SCS.jl. Part of each iteration of the solver is solving a system of linear equations. Scs provides some freedom in this respect. i.e. one can choose one of the following provided methods: Direct Solver using qdldl and amd Indirect Solver using conjugated gradient Indirect Solver on gpu using sparse conjugated gradient through CUDA (You may also implement your own solver.
Jul 7, 2019 2 min read

Replication Details for 1812.03456

Below are replication details for computations with special linear groups as described in Section 5.1 of paper On property (T) for $\operatorname{Aut}(F_n)$ and $\operatorname{SL}_n$. The content of the accompanying jupyter notebook is reproduced below. For exact details of computations for $36(\operatorname{Adj}_5 + 2 \operatorname{Op}_5) - 50\Delta_5 \in I\operatorname{SAut}(F_5)$ see the document deposited with the dataset in zenodo data repository. Table of Contents Generating set Group Ring and Laplacians Orbit Decomposition Elements Adj and Op Optimization Problem Solving the problem Checking the solution Checking in interval arithmetic Installation The following instructions were prepared using julia-1.
Jan 17, 2019 18 min read

Replication Details for 1712.07167

This post documents replication details for paper $\operatorname{Aut}(\mathbb{F}_5)$ has property (T) by M. Kaluba, P.W. Nowak and N. Ozawa. The current version of replication details is located in Nextjournal docker container. The following notes are exported from there. Table of Contents Getting julia project Running tests (optional) Getting the pre-computed data Recomputing from scratch group ring structure Loading the solution Certification Setting up the environment The code below is designed to run on julia 1.
Dec 21, 2017 4 min read

Replication details for 1703.09680

This post documents replication details for paper Certifying numerical estimates of spectral gaps by M. Kaluba, P.W. Nowak. This guide is rather outdated. All of the computations can be done (and much more effectively) by exploiting the symmetry of the Laplacian as is described in Replication Details for 1712.07167. For more details on the method see $\operatorname{Aut}(\mathbb{F}_5)$ has property (T). Table of Contents Installing To run the code You need julia-v0.
Mar 28, 2017 3 min read

Contact